When Einstein Walked with Godel: Excursions to the Edge of Thought is a collection of 24 full length essays plus several shorter pieces written by Jim Holt, the author of the thought-provoking 2013 book Why Does the World Exist? Holt discusses a wide range of subjects that relate to at least one of three categories: "our most general conception of the world (metaphysics), how we come to obtain and justify our knowledge (epistemology), and even on how we conduct our lives (ethics)" (p. x).
When Einstein Walked with Godel: Excursions to the Edge of Thought is a fascinating book. I will not review each essay, but I will single out a few of my favorites to whet your appetite to read them all.
The book begins with the title essay. It is a fun fact that two of the greatest geniuses of all-time regularly took walks together, and it is touching that Einstein once said that he went to his office at the Institute for Advanced Study "just to have the privilege of walking home with Kurt Godel." I don't take Einstein's statement as false modesty--like most, if not all, geniuses, Einstein was quite aware of his capabilities--but rather as a genuine statement of affection and admiration for his friend Godel.
Einstein and Godel were not only geniuses but also--regardless of the fame and acclaim that both received--outsiders. Holt notes that after Einstein initially failed to receive his doctorate in physics he gave up on pursuing an academic career, declaring "the whole comedy has become boring." Einstein worked as a patent clerk, but he followed closely the scientific questions of the time, and he read with interest about what Henri Poincare termed the three greatest unsolved questions: (1) the photoelectric effect, (2) Brownian motion, and (3) the nature of the "luminiferous ether" which had yet to be detected experimentally but was supposed to be the medium through which light waves traveled. Holt explains, "Working alone, apart from the scientific community, the unknown junior clerk rapidly managed to dispatch all three" questions in a series of papers published in 1905, when Einstein was just 26 years old (p. 5). Einstein received the 1921 Nobel Prize in physics for his work on the photoelectric effect, but his equation E=MC2 forever transformed our understanding of space, time, and energy.
Einstein had already finished most of the work for which he would be most remembered prior to Godel's birth: "In 1906, the year after Einstein's annus mirabilis, Kurt Godel was born in the city of Brno (now in the Czech Republic). Kurt was both an inquisitive child--his parents and brother gave him the nickname Herr Warum, 'Mr Why?'--and a nervous one. At the age of five, he seems to have suffered a mild anxiety neurosis. At eight, he had a terrible bout of rheumatic fever, which left him with the lifelong conviction that his heart had been fatally damaged" (p. 8).
Godel developed two incompleteness theorems that had profound implications for mathematics and epistemology. The first incompleteness theorem is that no logical system can capture all the truths of mathematics; within any mathematical system there will always be at least one statement that cannot be proven or disproven within that system. The second incompleteness theorem is that no mathematical system can be proven, within its own devices, to be free from inconsistency. When Godel first presented his theorems, few people understood them, and some of the people who understood vigorously objected because they disliked the implications about the nature and limitations of knowledge.
The nature of time is one of the subjects that Einstein and Godel discussed. For Einstein's 70th birthday, Godel presented to him a gift: a solution to Einstein's General Relativity equations demonstrating the theoretical possibility of time travel in a rotating universe. Einstein did not like the idea of a rotating universe in which time travel is possible, but he found no flaws in Godel's math. Holt notes that Godel presented the solution as proof that, in Holt's words, "time itself is impossible. A past that can be revisited has not really passed. And the fact that the universe is expanding, rather than rotating, is irrelevant. Time, like God, is either necessary or nothing; if it disappears in one possible universe, it is undetermined in every possible universe, including our own" (p. 13).
The unanswered and perhaps unanswerable question is that if time is impossible then why do we feel its passage so intensely and so vividly? That question leads to other unanswered and perhaps unanswerable questions. Is everything that we consider to be real and to be meaningful an illusion, or a collective delusion/hallucination? If so, what is the purpose of such a grand deception?
Einstein and Godel could not answer those questions, but that did not stop them from walking, talking, and thinking together.
"The Riemann Zeta Conjecture and the Laughter of the Primes" begins with a discussion of the "Copernican Principle": the "Copernican Principle," first formalized as a mathematical equation by astrophysicist J. Richard Gott III in 1993, postulates that at any given time we are not privileged observers of an event, which in simple terms means that when you see something you are not in the first 2.5% of observers and you are not in the final 2.5% of observers. In other words (to borrow Holt's example from pp. 36-37), if you are attending a play that has had n showings then there is a 95% chance that the play will have no more than 39 x n showings and a 95% chance that the play will have no fewer than n/39 showings. Something that has already existed for a long time is statistically more likely to continue to exist for a long time than something that has only existed for a short time.
Holt demonstrates that laughter and numbers are things that we share with species who have a common ancestor with us several million years ago. Since laughter and numbers have already existed for millions of years, based on the "Copernican Principle" it is more likely than not that laughter and numbers will exist one million years from now.
What is the Riemann Zeta Conjecture and what does it have to do with any of this? The Riemann Zeta Conjecture is a hypothesis about prime numbers. If Bernhard Riemann was correct, then prime numbers have a hidden harmony, but if he was not correct then the provisional proofs of thousands of theorems "conditioned" on the correctness of his conjecture will be falsified and, as Holt puts it, "the part of higher mathematics that is built upon it will collapse" (p. 43). In layman's terms, the distribution of prime numbers seems at first sight to be random, but Riemann suggested that when one considers the full (i.e., infinite) set of prime numbers then order emerges from what looks to be chaos. The Riemann Zeta Conjecture has been one of the great unsolved math problems for more than 160 years. Applying the Copernican Principle, Holt suggests that there is a 95% chance that it will be solved within the next six millenia. Holt further argues that once the secret of prime numbers is understood then mathematics will be revealed to be, as Bertrand Russell proposed, "as trivial as the statement that a four-footed animal is an animal." Or, put another way, Holt believes that laughter has a better chance of being an integral part of human existence a million years from now that mathematics does. One could object that his opinion is based too much on speculation, but if you read the entire chapter you will be intrigued even if you remain skeptical.
In "Benoit Mandelbrot and the Discovery of Fractals," Holt, in his
typical engaging style, describes Mandelbrot's eventful life, and
Mandelbrot's inquisitive spirit that enabled him to find order where
others saw only disorder and chaos. Benoit Mandelbrot discovered the Mandelbrot set, and demonstrated that things that had been thought to be rough or chaotic--such as clouds and shorelines--actually have a "degree of order." Mandelbrot coined the word "fractal" (derived from the Latin word for broken) to describe self-similar forms, phenomena that are visible not only in physical objects such as clouds and shorelines but also in the behavior of financial markets. Orthodox thinkers in both mathematics and economics bristled at Mandelbrot's ideas but Mandelbrot was stubborn and undeterred.
Academic tenure eluded Mandelbrot--or he eluded it--until he was 75, but his unorthodox way of thinking about mathematics found a home for many years at, of all places, IBM; for a lengthy period of time, the computer manufacturing giant decided to fund pure research. "We can easily afford a few great scientists during their own thing," the director of research told Mandelbrot (p. 98), and those words were music to Mandelbrot's ears. He worked for IBM from the late 1950s until 1987, when IBM decided it no longer wanted to pay great scientists to do their own thing. Six years later, IBM posted $8 billion in losses, at the time the largest such total in U.S. corporate history. Did IBM stop funding geniuses because it ran out of money, or did it run out of money because it stopped funding geniuses?
Fractals are not only beautiful to observe, but they exist at the nexus of science, math, and art. Here is an example of a fractal:
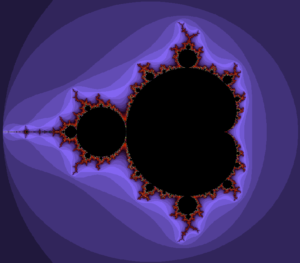
"The Ada Perplex: Was Byron's Daughter The First Coder?" methodically debunks attempts to elevate Lord Byron's daughter Ava from a person who struggled to learn basic mathematics to someone who supposedly was a pioneering scientific figure. It is important to give proper credit to anyone--man or woman--whose accomplishments merit recognition, but it is dishonest and harmful to rewrite history for the purpose of heaping honor on an individual who is not worthy of such recognition. Holt's effort to set the record straight is important, and hopefully will not only result in a widespread reassessment of Lord Byron's daughter but also inspire a thorough and thoughtful examination of other historical figures who are given too much--or too little--credit.
Holt's curiosity, smooth writing style, and active engagement with any subject that he tackles would probably enable him to communicate engagingly even about uninteresting subject matter, but it is a treat to read his writing about the fascinating subjects briefly described above, as well as the other topics addressed in When Einstein Walked with Godel: Excursions to the Edge of Thought.
Further Reading:
Albert Einstein the Man (December 13, 2015)
Kurt Godel: Mathematician/Philosopher Extraordinaire (August 25, 2016)

No comments:
Post a Comment